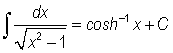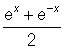Proofs and Derivations
The Derivative of the Inverse of sinh x

The Derivative of Cosh -1 x
 

Let y = cosh -1 x
 

We have no rules that will allow us to differentiate this function, so we are going to first write it into another form, so that we can apply differentiation rules that we have developed.

Apply the function cosh x to both sides of this equation, to obtain
 
 
 

cosh y = x


 

Rewrite this as,
 
 

x = cosh y.




 

Since cosh x = 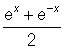, we may rewrite x = cosh y as
 
 

x =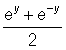 



 

Multiply both sides of this equation by '2' and then flip the equation to obtain
 
 
 

= 2x.



 

Now, multiply both sides of the above equation by 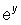, so the equation becomes
 
 
 



 

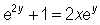
and this may rewritten as
 
 

.
 
 

Now, we may seem to have created a mess, but we will soon be able to solve this equation for y and obtain a form that we can differentiate.

We now let  z = 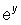, so that the above equation turns into a quadratic equation in 'z'
 
 
 

z2 - 2xz + 1 = 0.



 

We can solve this equation for z (using   ?    method) and obtain
 
 
 


 

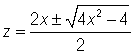
which simplifies to
 
 
 

.



 

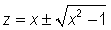We choose the positive root, so that we have a function of x on the right side of the equation below,
 
 
 

.



 

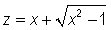Now replace z with 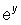and rewrite the above equation as
 
 
 

.



 

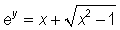Apply the natural logarithmic function (ln x) to both sides of this equation to obtain,
 
 
 

.



 

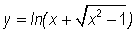We have now achieved our first goal of rewriting the function y = cosh -1x in a form which can be differentiated.
 

So, let's differentiate the above equation. We get
 
 
 



 

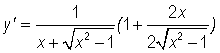
which simplifies to
 
 
 



 

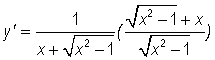
and further simplifies to
 
 

.



 

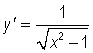We have now completed this derivation, as we now know that
 
 
 


 

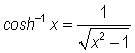
which has the integral form